Relationship to other projects/themes
WP 4.1 is the main tool used in WP 3.1 and by extension WP 3.2 for developing a new theoretical basis for nonlinear modal analysis.
It is also being used in WP 1.2 for identification methods. Extensions to random systems via WP 2.1 are also being explored. WP 4.3 on rotating structures has more of an industrial focus, and is linked to the application parts of WP 3.2.
The initial work carried out in this area had a strong overlap with the work on energy harvesting —Themes 2 and 5.
Aims
To apply bifurcation and normal form theory to multi-degree-of-freedom structural dynamics applications.
In particular, to study rotating structures.
Progress to date
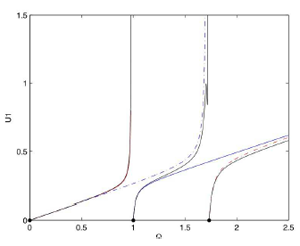
Figure 1: Backbone curves from a 3 degree-of-freedom coupled system with a purely cubic nonlinearity associated with a nonlinear energy sink. U1 is the amplitude of the first mode, and Ω is the response frequency.
The work originally planned for normal form theory in WP 4.1 was been completed at the end of month 23. During this Milestone MS2 was completed by developing extended normal form transformations to simplify multi-degree-of-freedom nonlinear systems. Milestone MS2 required a normal form method that could be applied to systems and used eventually used for modal testing.
The first stage was to clarify how modal decomposition could be achieved using normal forms, and this work was carried out initially by Andrea Cammarano jointly with Tom Hill working on WP 3.1 The key results focused on how internal resonances could be dealt with via the normal form process, are publications are listed under WP 3.1.
The key Deliverables for MS2 were the work on modal decomposition Neild et. al. (2013) and Hill et.al. (2014)a, then the use of backbone curves via the normal form method Cammarano et. al. (2014)b. In addition, the overall normal form approach developed in WP 4.1 was recently disseminated in the research text [1]. Further to the work on a theoretical framework for modal testing in collaboration with WP 3.1, two further directions have developed from the initial work undertaken.
First the use of machine learning techniques to identify normal forms from data has been investigated in collaboration with WP 1.2 — as already reported. Second PhD student Xuanang Liu has been working on normal form transformation for systems with higher numbers of degrees-of-freedom, and continuous systems. The results from studying an example 3 degree-of-freedom systems is shown in Figure 1. This shows a 3 degree-of-freedom coupled system with a purely cubic nonlinearity associated with a nonlinear energy sink. U1 is the amplitude of the first mode, and Ω is the response frequency.
More detailed results are to be presented at the forthcoming IMAC XXXIII conference [2]. In addition investigators Neild, Champneys & Wagg have been working on classifying the new normal form techniques in the overall context of classical approaches. This has led to [3] and a further paper on the structure of the associated homological operators is in preparation. For WP 4.3 the early work focused on an energy harvesting cross-over with Themes 2 and 5, leading to paper [4]. The work was then refocused on the industrial problem of drill string dynamics.
More specifically, a major concern within the oil drilling industry remains the high risk associated with the drilling bit and tool failure from the build-up of damaging vibrations. The work was then refocused on the industrial problem of drillstring dynamics.
More specifically, a major concern within the oil drilling industry remains the high risk associated with the drilling bit and tool failure from the build-up of damaging vibrations. Effective understanding of the drillstring dynamics is essential for efficient drilling operation. Complex dynamic behaviour is often observed in the drillstring due to friction, impact, unbalance eccentricity and energy exchange between different modes of vibrations.
The interaction between the drillstring and borehole wall involves nonlinearities in the form of friction and contact. In order to tackle this problem, a multiple disk model has been developed to study the behaviour of the system. The effects of impact, friction and mass unbalance are included in the model. The drillstring borehole interaction induces whirling behaviour of the drillstring causing forward whirl, backward whirl or intermittent bouncing behaviour depending on the system parameters.

Figure 2: Variation in the behaviour of the drillstring was observed at different stabilizer location.
A critical steady state behaviour within the system is the backward whirling of the drillstring, which reduces the fatigue life of the drillstring. A two disc theoretical model was developed in order to analyse the influence of different parameters, such as the eccentricity and rotational speed, on the induced backward whirl within the system [6]. The influence of rotor speed on the system dynamics is explored using run up and run down.
The model was extended to multiple disks to analyse the influence of axial loading on the whirling [7]. The actual drillstring is asymmetric with the lighter drillpipe forming the upper section and heavier drill collar forming the bottom section which applies weight on the bit.
It was found that axial force changes from tension to compression along the drillstring. Initiating the system in its first mode, near the primary resonance frequency, a transition in behaviour along the length of the drillstring is observed due to the axial load and bending moment interactions, as shown in Figure 2.
The plan is to further extend the bifurcation analysis of multi-disc drill string models using continuation methods. Progress will also be made towards parameters and models that more closely match the physical system. The possibility of model validation, perhaps with a new experimental test rig, will be investigated.